Clicker Questions
Our experiences with using clicker questions in introductory physics courses (and the research that supports their effectiveness) have motivated us to apply these education principles to more advanced courses, most important being the idea that students will learn and retain more when they are active participants in the classroom. We explain here how the clicker questions in this package are organized, and the kinds of information contained in the accompanying notes (from the instructors who created/used them, and the learning assistants who facilitated student discussions during the FA11 semester). We also provide some motivation for adopting the use of clicker questions (and other active engagement techniques) in upper-division classrooms, and offer some suggestions about how they may be put to effective use. A plethora of information on the use of clickers in science classrooms of all kinds can be found at: http://stemclickers.colorado.edu. You can also download a PDF version of this guide to the electrodynamics clicker questions.
| I.Organization of Clicker Questions |
| II. Notes on Clicker Use |
| A.Why use clicker questions in upper-division physics courses? |
| B.How can clicker questions be used effectively in advanced courses? |
I. Organization of Clicker QuestionsThese electrodynamics clicker questions can be downloaded with the links at the above left, which are organized into topics according to their order of presentation in Griffiths: 0 – Review Topics (Griffiths, Ch. 1-6) 1 – Electrodynamics and Maxwell’s Equations (Griffiths, Ch. 7) 2 – Complex Exponentials and AC Circuits (not covered in Griffiths) 3 – Conservation Laws (Griffiths, Ch. 8) 4 – Electromagnetic Waves (Griffiths, Ch. 9) 5 – Potentials and Fields (Griffiths, Ch. 10) 6 – Radiation (Griffiths, Ch. 11) 7 – Special Relativity (Griffiths, Ch. 12) |
The questions are in the form of PowerPoint slides, but because there are sometimes compatibility issues between Macs and PC’s (equations or images may become distorted), PDF versions are also available. Additional information on each question can be found in the “notes” section of each PowerPoint slide. This generally includes the final distribution of student responses when they were used, and commentary from those who wrote/used them. As much as possible, we have tried to address when and why a question was used, the kinds of student questions/responses they inspired, and specific examples of difficulties they had, or the kinds of reasoning they invoked in their answers.
II. Notes on Clicker Use
A. Why use clicker questions in upper-division physics courses?
Many consider a 1998 paper by Richard Hake to be a wake-up call to instructors that traditional methods of teaching (lectures from a blackboard, sometimes interspersed with demonstrations) are not effective for most students in promoting a more-than-superficial understanding of physics.[1] Hake classified a number of introductory level physics courses (from various institutions) according to whether they had been taught in a traditional manner, or had used active engagement techniques to some degree or other. Students were given the Force Concept Inventory (FCI) at both pre- and post-instruction, which basically tests whether they have mastered Newton’s three laws of motion. Results were reported in terms of a “learning gain” <g>, as a measure of how much the average student learned in that course, relative to how much they had yet to learn when starting out. Students from different types of schools (e.g., public vs. private) may vary in the amount of physics they already know coming into the course, which is taken into account by using a relative learning gain when making cross-institutional comparisons on the effectiveness of instruction.
For the courses classified as “traditional” lecture environments, the learning gains were remarkably low, with the majority of them falling into the 20-30% range. Roughly speaking, this means that most students were only learning about one quarter of what they didn’t already know at the outset, at least as far as Newton’s laws are concerned. Although the classes using active engagement sometimes scored similarly low gains, the majority of them scored learning gains from two to three times higher than the average traditional course. This result has been replicated in other studies,[2] which also found that long-term retention of conceptual understanding in E&M is augmented by the use of interactive tutorial activities in the place of traditional recitations.[3] This tells us that active engagement is important for promoting student learning at the introductory level, but the choices instructors make during implementation make a difference.
With regard to upper-division courses, an interesting comparison is made by Pollock[4] of pre- and post-instruction scores of junior-level electromagnetism students on the Brief Electricity and Magnetism Assessment (BEMA), which focuses on fundamental concepts from introductory E&M. He found there to be no significant improvement in student BEMA scores after having completed a traditional advanced E&M course, implying that the topics were taught in a way that doesn’t help to solidify their understanding of the most basic concepts, which instructors typically assume these advanced students have already mastered. If students have not mastered these fundamentals, it calls into question whether they can have a deep understanding of more advanced electrodynamics topics.
When active engagement has been shown to be effective for promoting student learning at the introductory level, why not apply these techniques to more advanced courses? It is important to acknowledge the different perspectives instructors have on what makes upper-division courses different from introductory courses. The most obvious difference is that the topics themselves are more advanced, and are generally developed using a more abstract mathematical formalism. Upper-division students have already demonstrated some amount of aptitude for learning physics by passing into the advanced courses, whereas introductory courses typically serve a more mixed population. Upper-division courses are more often held in smaller classrooms, which affords students more opportunities to ask questions and engage in discussions. Many faculty also perceive student success in upper-division electrodynamics as an indicator of overall potential as a future physicist; instructors often feel they should be preparing the more advanced students for future graduate study, and that average students will learn as best they can. Physicists often approach upper-division courses in a way that mirrors how they themselves learned as undergraduates, and can have difficulty imagining that it could or should be done any other way.
In other words, active engagement techniques are often associated (among both instructors and students) with introductory learning, and there is a belief that the very nature of more advanced topics requires a more traditional presentation. However, we know that many of our advanced students are not learning as much or as well as we would like,[5] and we know that active engagement is a sound principle for improving learning and understanding in introductory courses. Even in smaller classrooms, students may not realize they are confused by a specific topic (or even know what question to ask) until given the opportunity to apply what they have learned, and clicker questions offer both instructors and students a chance to gauge their level of understanding during the lecture. We believe scientific argumentation (both oral and written) is a skill that is developed through practice, and that important learning is taking place as students articulate their understanding to each other, and defend their beliefs against alternative conceptions.
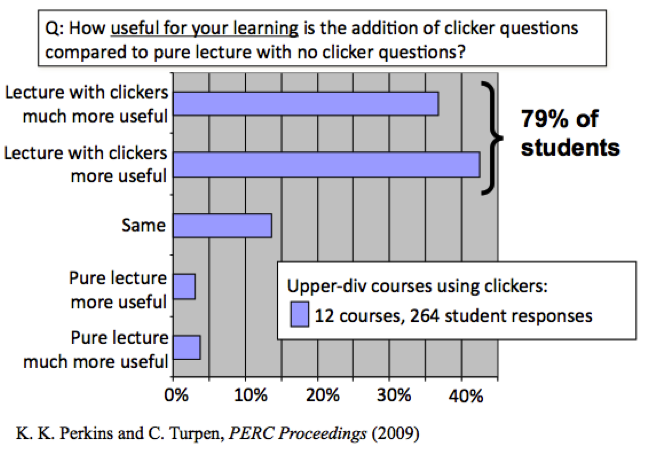
Perhaps most important is the reaction from students to the use of active engagement in upper-division courses, which has been overwhelmingly positive. A post-instruction survey was given to students in a number of advanced physics courses at CU on their perceptions of clicker use in upper-division classrooms.[6] Four out of five students who have experienced using clicker questions in an upper-division course would say that augmenting lectures with clicker questions is more useful for their learning than pure lectures without clickers, and around 15% responded neutrally, essentially saying that it didn’t take away from their learning experience [see figure below].
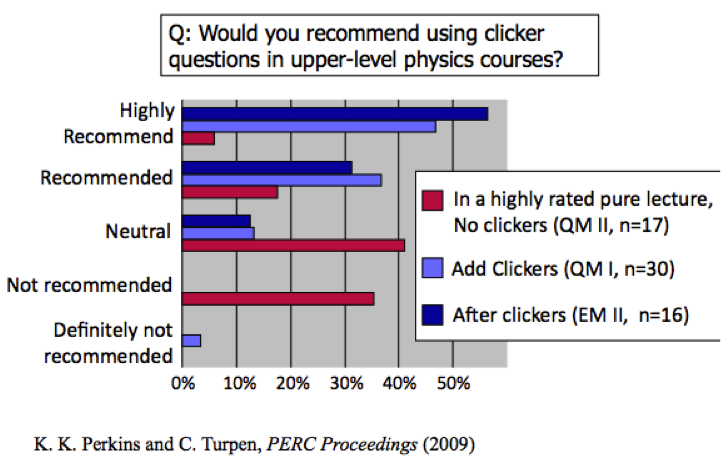
Just as interesting is the fact that, as with many instructors, students who had never used clickers in an upper-division course were skeptical about their usefulness in that context. Students were asked following a popular pure-lecture course on quantum mechanics if they would recommend using clickers in advanced courses, with the majority responding either neutrally or not recommending their use. [See figure below.] In contrast, most students from a quantum mechanics course that had used clickers during lecture would recommend using them in advanced courses. Students who had used clickers in a first-semester electromagnetism course continued to favor their use after taking the second-semester course (E&M II), where they were not used.
B. How can clicker questions be used effectively in advanced courses?
Having motivated the use of clicker questions in upper-division courses, a natural question is: how can they be effectively incorporated into lectures? Active engagement doesn’t require instructors to give up lecturing entirely, and clicker questions can be judiciously inserted into a lecture at key points for a number of purposes, for example: to check for conceptual understanding, to have students apply a concept to a new situation, to underscore an important idea in a long derivation, or to help them make connections between a physical system and the mathematics used to describe it. Specific examples of these are given below.
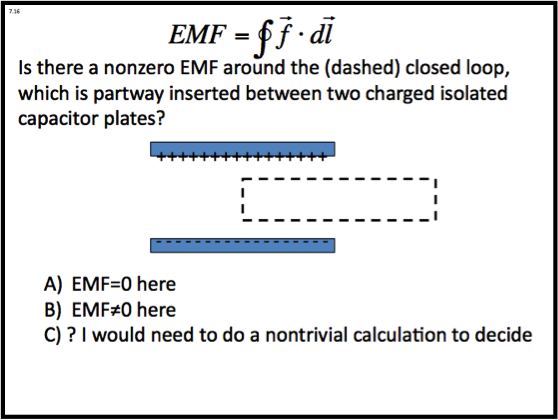
Clicker questions are often only associated with testing conceptual understanding – asking students to come up with an answer without resorting to mathematical calculations. The sample question below asks whether the electrostatic field of a charged capacitor plate is capable of generating a non-zero EMF around a closed loop. This question was used in two separate electrodynamics courses at CU, and in both cases ~25% of students got this wrong; when asked, many of them intuitively knew that a charged capacitor shouldn’t be able to drive a current around a loop, but some weren’t considering the existence of fringing fields, or couldn’t visualize how they were causing the line integral to vanish.
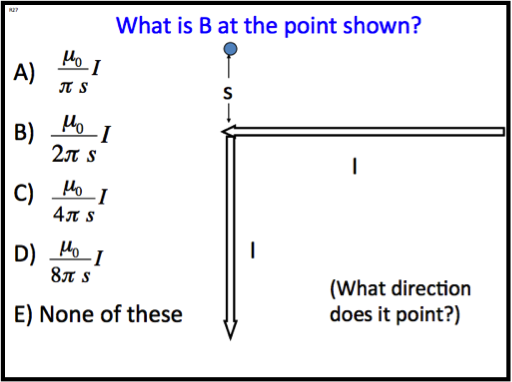
Students can also test their understanding of important concepts by applying newly derived results to a novel situation. In one of our classes, following a standard derivation of the magnetic field due to a long, straight wire using Ampere’s law, students were asked in the following question about the magnetic field at a point near a long wire that takes a 90 degree turn. Answering this question requires students to consider the role of symmetry in deriving the answer for the straight wire, and also the directional variation in the field produced by a section of current according to the Biot-Savart law. Because the vertical section contributes nothing to the field at point s, the field can be found by simply dividing the expression for a straight wire by half. Most students initially got this question wrong.
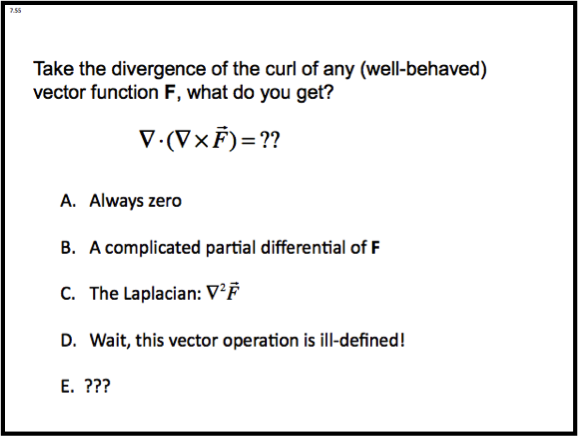
Sometimes there is an essential point that students must keep in mind in order to follow along with an important derivation. When discussing Maxwell’s modification to Ampere’s law, arguments involving the continuity equation required students to know that the divergence of the curl of a field is identically zero. The question below was used to underscore the importance of this point in the derivation, and to be sure that students realized this was a general result, and not specific to any particular type of field.
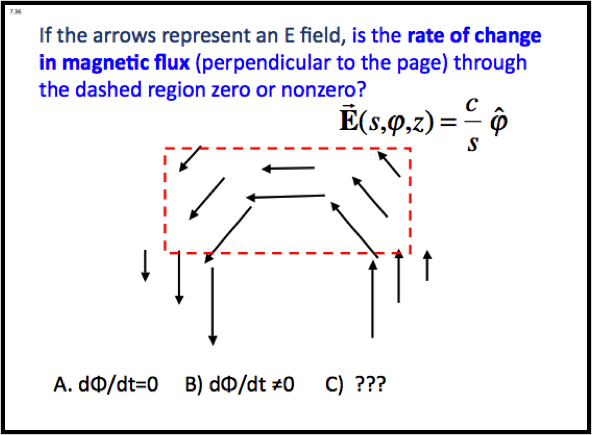
Clicker questions can also be used to help students make a connection between a physical situation and the mathematics used to describe it. A mathematical formula for the electric field is required to answer the question below, which essentially asks them to relate the curl of an electric field to the rate of change of magnetic flux in that region. Even though the field may appear “curly” to students, the 1/distance dependence of the field actually makes its curl everywhere zero except at the origin. This answer can be gotten without looking at the explicit expression for the curl of a field in cylindrical coordinates, by using a specific line-integral as an example, where the increase in arc length along a curve at a greater distance from the origin exactly cancels the diminished magnitude of the field. Before discussion, students in the FA11 and SP12 E&M II classes at CU were evenly split between the curl being zero (A) and non-zero (B).