This manual supercedes all previous versions of instruction for Physics 2020 experiments. Earlier versions should not be used as they are now obsolete.
 
 

General Information.

These notes contain instructions for six experiments, which you will perform according to the schedule distributed in the first lecture. For each experiment, you are to complete the write-up during the 2 hour class period and hand in your lab notebook at the end of the period. In order to complete your lab on time, it is essential that you study the instructions for each experiment before the start of the laboratory session. To encourage you to study the lab instructions, there are a set of questions with each lab, on a separate question sheet, that you are to answer and hand in at the beginning of your lab period.

You will generally work with one or two lab partners. Although your data will be the same as that of your partners, and although you may freely discuss the physics of the experiment and compare final answers with your partners, you must still record all data, do all calculations, and answer all questions in your own lab notebook.

Your lab notebook should be the sort with ruled squares on every page. On the front of your lab book, write your name, section number, and your lab instructor's name. Your name and section number should also be on the bottom edge of the book, so that it can be quickly located in a pile of books. Put a table of contents on the first page. When doing the lab, write only on the right hand page of your notebook, since this leaves room for your instructor's comments and for your use in emergencies. Always leave yourself plenty of room. Start each report on a new page.

Instructions for writing reports

Since you have only two hours to do the lab and write the report, you are not required to write out any detailed introduction or description of procedures. Your report should contain your data, your calculations, and the answers to any questions in the lab instructions, and brief conclusions. Of course, if you have a pertinent comment to make, perhaps about something unusual or unexpected in the experiment, you are encouraged to write it in your report. In writing your lab report, follow these guidelines:

1. Always write in ink in your lab notebook. Cross out mistakes. You can even cross out whole pages and start over if you wish. Never tear out pages and never write anything on loose sheets. The whole idea of a lab notebook is that it is a permanent record of all activities in the lab.

2. Number each page and write the date at the start of your report. Give the title of the lab and the names of your lab partners. The lab notebook should be thought of as a diary.

3. Define all symbols used. A short phrase or drawing is good. Be sure to use the same symbols that are used in the laboratory instructions.

4. Label the various parts of your report so that the reader can follow your work. Examples: "Part 2, Inelastic Collisions", "Calculation of the moment of inertia I", "Answer to question 1."

5. The best way to display lots of data is with a table. Be sure your table has clearly labeled column headings with the units shown.

6. In general, you should report the uncertainties on measured quantities. Uncertainties should be written with only 1 significant figure, very occasionally 2, but never 3. Example: m = 1.777 ± 0.002 kg or q = 1.600 ± 0.015. Never: y = 32.281 ± 0.333 m. (See the section on uncertainties and significant figures below.) If you do not explicitly state the uncertainty, it is always assumed that the uncertainty is about ± 1 or 2 in the last place shown.

7. Graphs should have a title and clearly labeled axes with units shown. Example of a title: "Velocity vs. time for a freely falling weight".

8. Always display the correct number of significant figures on your final answers. Never include useless extra digits on calculated quantities. In intermediate calculations, it is OK to keep 1 or 2 extra significant figures to avoid round-off error.

9. Always include units on numbers. Sometimes you can leave out units in intermediate calculations, if it is clear what you are doing. (See the example below)

10. Always write down an equation using symbols before inserting numbers. An equation like [] is useless because the reader does not know what the numbers 5.42 and 2.33 represent. It should be written like:

For example, a good note book might contain the following:

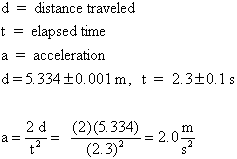
Notice that the symbols are defined, the equation was written with symbols first and then number were inserted, and the final answer has units. It is OK to leave out units in the intermediate step, if it is clear to the reader that you are using a consistent system of units such as the SI system (also called the MKS system, meter-kilogram-second.). Also note that the final answer has 2 significant figures, because the least precise input number (t = 2.3 s) has only 2. (More on significant figures below.)
 

Uncertainties in measured quantities

In science and engineering, a measured quantity is never known exactly. There is always some uncertainty in the measured value. For instance, suppose you are considering buying a big, new refrigerator, but you are afraid that it may not fit through the door of your house. The manufacturer says that the fridge is exactly 87.5 cm wide; you measure the width of your doorway with a meter stick, and you get 87.7 cm. It looks like you can get that big fridge inside. Except you might have made an error in the measurement because the end of the meter stick was worn off a little. Or maybe it was difficult to get the stick really close to the edge of the door because something was in the way. Or maybe the stick looks old and you suspect that it is warped and inaccurate. Perhaps the doorway does not have a uniform width: near the top of the door, it is 88.0 cm wide while near the floor it's 87.4 cm. With a little experience in making measurements, you might decide that the width of the door is 87.7 ± 0.4 cm. In this case, the uncertainty is 0.4 cm. That is, it is very likely that the doorway is no larger than 87.7 + 0.4 cm and no smaller than 87.7 - 0.4 cm, but you cannot be more precise until you make a better measurement. So, when the uncertainty is considered, you do not know whether the fridge will fit through the doorway.

We represent the uncertainty in some quantity x with the symbol dx. (d is the Greek letter delta. dx is read "delta x".) By convention, the uncertainty dx is always positive and the value of the quantity is x ±dx. In this course, whenever you measure something, you should estimate the uncertainty. A good general rule is this: If you make a careful measurement under good conditions, the uncertainty is usually the smallest division on the measuring device. For instance, if you measure the width of a refrigerator with a meter stick that reads to millimeters, then the uncertainty in the measured width W is dW = 1 mm. (W = 87.5 ± 0.1 cm) If you weigh something on a balance and the smallest division on the mass scale is 0.1 gram, then the uncertainty of the mass M is dM = 0.1 g. (M = 52.3 ± 0.1 gram) You might be tempted to interpolate between the smallest divisions and estimate the uncertainty to be smaller than 1 division. But this is usually a bad idea because if the scale was really accurate to better than 1 division, then the manufacturer would have put finer divisions on the scale. Remember, just because you can read the scale to a little better than 1 division does not mean that it is accurate to better than one division.

Of course, measuring conditions might be less than ideal. It might be impossible to get the meter stick close to the edge of the measured thing, or the thing might be moving while you are trying to measure it. In these cases the uncertainty is larger than the smallest division. One way to estimate the uncertainty is to have two people make independent measurements of the same thing and then compare their numbers.

IMPORTANT: In this course, if you do not state the uncertainty, we will assume that the uncertainty is about ± 1 to ± 3 in the last place. So, if you measure some length to be 1300 ± 1mm, you can report it as 1.300 m (not 1.3 m). There's a big difference between 1 m and 1.000 m!
 
 

Significant Figures

The number of significant figures (sig. figs) in a number is the number of physically meaningful digits, not including zeroes to the left. The numbers 12.0, 511, and 0.00321 all have three significant figures. You don't count zeroes to the left because the number of such zeroes depends on the units used. For instance, 2.3 cm = 0.023 m = 0.000023 km (kilometers) - these all have 2 significant figures. On the other hand, zeroes on the right are vitally important when counting significant figures. Suppose you measure the width of a desk with a meter stick and you find that it is exactly 1500 mm - as nearly as you can tell. If you give the answer in meters, you should write it as 1.500 m (not 1.5 m).

Here are important things to know about using sig. figs:

Often, in experimental work, you measure several quantities, and then calculate some other quantity, based on your measurements. The subject of error analysis describes how to determine the uncertainty of the calculated quantity, given the known uncertainties of the measured quantities. In general, this is a difficult problem. In this course, instead of computing the uncertainty, you will very roughly indicate the uncertainty by writing the final answer using the correct number of significant figures.

Often, the uncertainty of a calculated quantity can be roughly determined by simply counting significant figures. For problems involving multiplication and division and most other operations, except addition and subtraction, the rule is: the final answer should not have more significant figures that the least precise input number. Here, "least precise" means the number with the fewest significant figures. For example, suppose

(all with uncertainties of ± 1 in the last place) and suppose we want the value of

In this case, the number a is the least precise input number with only 2 sig. figs. So the answer is

The final answer should be rounded to 2 sig. figs.

This procedure of counting sig. figs. works well for multiplication or division or more complicated operations like tangents, exponential, etc., but it does not work for simple addition or subtraction. For addition or subtraction, the rule is: the answer should have the same decimal precision (10's or 1's or tenths or hundredths, etc.) as the input number with the least decimal precision.

For example,

In this case, x is known to about ± 0.01; its decimal precision is hundredths. But y is known to only ; its decimal precision is tenths. So the answer should have a decimal precision of tenths.

Another example: suppose a = 5321.2, b=5.1 (both with uncertainties of ± 0.1), then the sum a+b=5327.3. In this case, both input numbers, a and b, have a decimal precision of tenths, so the final answer should have a decimal precision of tenths, that is, an uncertainty of about ± 0.1. In this case of addition, you should definitely not round the final answer to 2 sig. figs, even though b has only two sig. figs.
 

To summarize:

For multiplication or division, the final answer should have the same number of sig. fig's as the input number with the least number of sig.fig's.

For addition or subtraction, the final answer should have the same decimal precision (tenths, hundredths, etc.) as the input number with the least precise decimal precision.

"Dry" Lab Report.

For these labs, we ask you to think carefully about what variables you will measure, what variables you will compute, and how you will display this information in your report.

Before the lab, we ask that you write out a "dry" lab report, that is, a report with no actual data, but which contains all the variables you will measure directly and all the variables that you will compute. The report should show the approximate format of the actual lab report, including tables and calculations. In this dry lab, give the symbols for all measured variables and a short description, in words, of the variables. For computed variables, give the equation for the variable and a short description of the variable, unless it was defined previously. For tables, give the column headings and show the general layout of the table.

There is more than one way to write a dry lab report. In general, it should show the variables, tables, and calculations in the same order that they will be done during the actual lab. Strive for a clear, clean, layout that the reader can follow easily. If a graph is called for in the lab, the dry lab should show a graph title, axes that are properly labeled, and a rough sketch of the expected shape of the graph.

We suggest that you make an extra copy of your dry lab, so that you can hand one copy in at the beginning of the lab, and use the other as a template for preparing your actual report during the lab.

Here is an example of a dry lab report. Suppose that the lab instructions describe an experiment in which the student measures the acceleration of gravity g by timing the fall of a marble over a measured distance L. g is then computed from equation . Two different lengths L are chosen and several measurements of t are made for each value of L. The average value of g is then compared with the known value, g = 9.80 m/s2. The dry lab for this part of the experiment might appear as follows.
 

Part 1: Determination of the acceleration of gravity g.

t = time of fall of marble

L = distance of fall of marble
 
 

Determination 1

L1 = ...
 

trial	t(sec)
1	..
2	..
3	..
4	..

t_avg = ....

Determination 2.

L2 = ...
 

trial	t(sec)
1	..
2	..
3	..
4	..

t_avg = ....

Comparison with g_known.

             g_known = 9.80 m/s²                 % discrepancy =  = ...