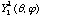Due Wed, Apr 26

(last hw, heck yeah!)

Required reading for this week: F+T Chapter 11.1, 11.2 (+11.3 for your cultural edification.) (Optional: Beiser through 6.7)

1. F+T 5-4

HINT: For the next 3 problems, note that F+T are asking for "spherically symmetric wave functions", which means that there is no dependence on angular variables, i.e. there is no angular momentum (i.e. l=0).

3. F+T 5-7a

4. F+T 5-8

5a) Verify that for the case l=1, m=1, the spherical harmonic function 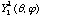(given in F+T table 11-2) is properly normalized.

b) Suppose that a bound-state energy eigenfunction has the form

where is a normalized spherical harmonic. What is the normalization condition on R(r)? Why?

Hint: see F+T problem 11-2

c) From F+T table 11-2, the spherical harmonic function for l=2, m=0 is .

Since m=0,. what can you say about the form of ?

Show that indeed satisfies the differential equation for ,

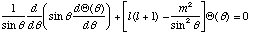(with l=2, m=0.)