Issued Wed, Jan 28 Due Wed, Feb 4

Required reading for this week: Finish Gas. Ch. 14, Start Gas. Ch. 15

1) In my class notes (pp 40-41 ) I derive the time evolution of the state of a particle precessing in a magnetic field , when it's initially in the particular (special) states and also .

a) Find <S_x>, <S_y>, and <S_z> (expectation values of the three components of spin) if the particle is initially in the state

b) If you measure S_x (once) at time t, what is the probability you will get + 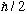? Same question for S_y and S_z.

c) Try to sketch, crudely/classically (or just describe in some intuitive way) how you picture the behaviour from part a. (Can you somehow try to draw a "classical picture" of S as a function of time?)

2) Below, I will refer to an "n"-SG, which means a Stern-Gerlach device measuring spin in the n direction (i.e. measuring ).

So, e.g., a "z"-SG measures 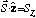, it's just a usual SG device measuring z component of spin.

The result of running spin 1/2 particles through an "n"-SG is always two separated beams, which you could call and , having .

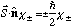(i.e. the output beams are "up" and "down" in the n direction)

For all parts below, you will be asked to answer two questions:

i) what is the quantum state of the output beam?

(give it in the form 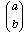, you just tell me what a and b are), and

ii) How many particles/sec are in the output beam?

The initial beam has 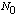particles/sec, all in the initial state .

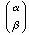(Problem continues on other side ->)a) The beam runs through a "z"-SG, but I block off the output beam with eigenvalue - . So only the + beam is output. (Answer i and ii above.)

b) Take the resulting beam from part a, and run it through an "x"-SG. Again, block the - 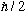beam, so only the + 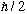beam is output. (Answer i and ii.)

c) Take the resulting beam from part b, and run it through a "z"-SG again. As usual, block off the - beam. (Answer i and ii.)

d) Now take the resulting beam from part c, and run it through an "n"-SG, where n is in the direction 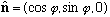. (Again, block off - ) (Answer i and ii.) Note for d: feel free to use the results Gas. derived on p. 243 and 244, especially Eq. 14-56.

3) Gas 14-14.

(This one is probably the hardest on this problem set. Be careful with your algebra, especially when you have to square sums of complex numbers!)

4) Gas 14-15.

Note! Gas asked you to find 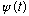for three different cases! (i.e, with initial state the eigenstate of for all three eigenvalues .)

But, I only want you to do the single case where the initial state is the eigenstate of with eigenvalue 0. Don't forget to normalize your wavefunctions...

________________________________________________________________

The last question is not for credit (so don't turn it in), but if you want to understand paramagnetic resonance, I recommend you do it:

Solve the equation

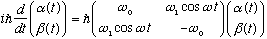
for , with given constants, and .

Show explicitly that ,

where

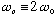
(This is precisely what I did in my notes, pp. 48-50. I skipped a few steps, which I want you to fill in. Even for the steps I did show, try to derive it all yourself. Just use my notes to help guide you if you get stuck...)