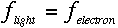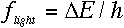Frequency and Energy
 The Bohr model and the Rutherford model give different predictions for the
frequencies of light that can be emitted by a hydrogen atom. In the classical
Rutherford picture, electromagnetic radiation is produced by orbiting
electrons, and the frequency of light emitted by a particular electron is the
same as the orbital frequency of that electron. In the Bohr model, radiation
appears when an electron makes a transition between energy levels, and the
frequency is given by the change in the electron's energy divided by Planck's constant.
The Bohr model and the Rutherford model give different predictions for the
frequencies of light that can be emitted by a hydrogen atom. In the classical
Rutherford picture, electromagnetic radiation is produced by orbiting
electrons, and the frequency of light emitted by a particular electron is the
same as the orbital frequency of that electron. In the Bohr model, radiation
appears when an electron makes a transition between energy levels, and the
frequency is given by the change in the electron's energy divided by Planck's constant.
These two formulas look quite unrelated. However, in the following proof, I'll show that Rutherford's equation becomes a good approximation to Bohr's at high energy levels.
Based on Balmer's formula,
Bohr assumed that the angular momentum L of an electron in a hydrogen atom had to satisfy the condition
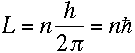
|
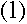
|
where n is a positive integer. If the electron is in a circular orbit
around the nucleus at the nth energy level, then its angular momentum
is also described by the equation
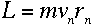
|
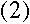
|
where m is the electron's mass and v and r are its speed
and orbital radius at this energy level. Therefore,
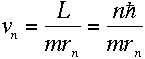
|
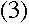
|
To find another relationship between r and v, we can apply
Newton's second law, F= ma, and the coulomb force, to the
electron--this has been done elsewhere.
Using Bohr's formula for the angular momentum, it turns out that
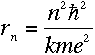
|
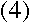
|
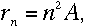
|
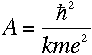
|
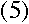
|
Then, plugging into equation (3),

|
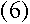
|
Now the total energy of an electron at a particular n can be calculated:
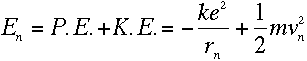
|

|
With the values obtained for r and v in (5)
and (6), this can be simplified to
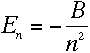
|

|
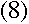
|
(B is related to the Rydberg constant, R.)
Given this value for E, it is clear that
the difference between energy levels n+1 and n
is

|

|
For large values of n, this energy difference approaches
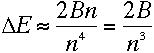
|
|
Now consider the orbital frequency of the electron. Its angular frequency at level n must be

|
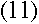
|
or, plugging in the values for r and v from equations (5) and (6),
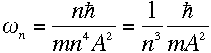
|
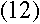
|
Using the value of A defined in equation (5),
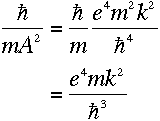
|
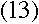
|
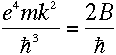
|

|
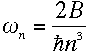
|

|
This bears a striking resemblance to the energy difference derived in equation
(10). And remarkably,
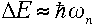
|
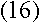
|
for large values of n. What's remarkable about it? Well, recall that
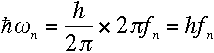
|
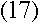
|
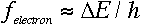
|

|
for large n, which makes Rutherford's and
Bohr's formulas approximately equivalent. Therefore, the frequency of the
emitted radiation approaches the electron's orbital frequency as n
increases.