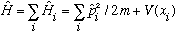
Physics 3220, Fall '97. Steve Pollock.
Here is the previous lecture
Back to the list of lectures
Last time we talked about the wave function for two identical particles. Here we generalize to N particles. Specifically, N non-interacting identical fermions in a potential well)
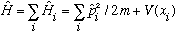
(Note that non-interacting means they don't notice each other's presence. So, the potential for particle i depends only on x_i, not on the location of the others.)
We want to solve
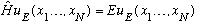
As always, we try separation of variables. In this case, that means we postulate (hope!) that we can write
 .
.
You take this form, plug it into the Schrodinger eqn, divide by u_E. Since the x_i's are all independent, we get N separate 1-D ordinary Schrod. Eq's!
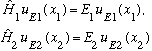
etc.
The total energy is simply given by
 ,
,
and all the H's are completely identical since we've assumed noninteracting particles (so all the H's are just K.E.(i)+V(x_i) ).
If the potential is one we've seen before, we know the solutions!
E.g., if we are putting N particles into an infinitely deep box, then
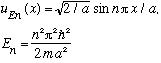
Finally, our total wavefunction would be
 .
.
Except, that's not quite right! This wavefunction satisfies the Schrodinger Eqn, and all the boundary conditions, but it is not antisymmetric!
Fortunately, our Hamiltonian is linear, so any linear combination of solutions is also still a solution! I claim that, (up to an overall phase), the correct answer is unique, and given by
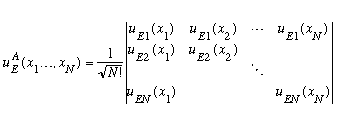
(This is called a Slater Determinant)
The determinant has just the right number and pattern of minus signs to ensure that this wavefunction is always antisymmetric under interchange of any pair of x's: exchanging particles i and j means you switch two columns of this determinant, which gives you a -1.
If you look at the Slater determinant, you can see that when x1=x2, two columns are identical, which means the determinant (and thus the total wavefunction) vanishes. Two particles cannot be in the same place! Also, if E1=E2, then two of the rows are identical, which means again the total wavefunction vanishes. So, you cannot have two identical fermions in the same place or in the same state! You may know this by the name Pauli Exclusion Principle. I would argue that this exclusion principle is just a simple consequence of the real Pauli principle, namely, that any quantum state involving identical fermions must be fully antisymmetric under particle interchange.
Of course, I have not been including spin in my description of the state. Since any E_n can have 2 spins, you can get 2 electrons with the same energy, but they'd have to be in a different spin state. So, in the Slater determinant, as we mentioned earlier, you should consider the E's (and the x's) to be somewhat more general than just energy and position, but should refer to the complete quantum state...
Summary: Identical fermions must always be put into a totally antisymmetric wavefunction, given by the Slater determinant.
Antisymmetry means that you will never find 2 electrons in exactly the same state, or exactly the same place.
Gasiorowicz goes on to discuss N fermions in an infinite box. You should read this! Let's look, instead, at a similar problem, N identical particles in a Harmonic Oscillator potential. Let's assume the particles do not interact with each other. (This last one is a big approximation, but is o.k. in a lot of real systems, including Wieman and Cornell's Bose condensate. )
What we do first is assume each particle has its own coordinate and energy, and then in the end simply antisymmetrize by hand.
Recall
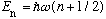 .
.
If the particles are bosons, and the temperature is low, the lowest state in the box will be with all N particles in the ground state. The total energy of the system will be
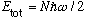 .
We call this state a "Bose condensate", because all the bosons have "condensed"
down into the same quantum state. The maximum energy for any individual
particle is
.
We call this state a "Bose condensate", because all the bosons have "condensed"
down into the same quantum state. The maximum energy for any individual
particle is
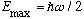 .
The wave function must be symmetric under interchange of any pair. (i.e. write
down the Slater determinant, only put in + signs between ever term!)
.
The wave function must be symmetric under interchange of any pair. (i.e. write
down the Slater determinant, only put in + signs between ever term!)
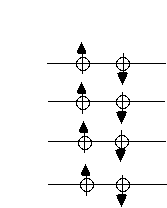
For fermions, the story is very different. The fermions can only go 2 to each energy level (because they have spin 1/2). The highest energy fermion has energy called the Fermi energy, given by
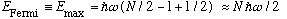 .
.
The total energy will then be
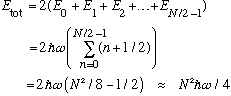
(Which is much larger than the Bose case, it goes as N^2, not N)
This property of fermions has tremendous implications. All of chemistry, nuclear structure, the properties of neutron stars, and more, arises fundamentally from this fact that fermions can't pile into the same state.
In an infinite square well, the above formula is replaced with
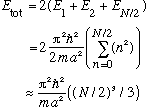
(Growing now as N^3), and the top energy is
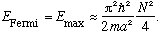
| 3220 main page | Prof. Pollock's page. | Physics Dep't | Send comments |