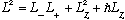Physics 3220, Fall '97. Steve Pollock.

Gas. Ch. 11 - Angular Momentum

3220 - Notes, lecture 38 (Fri, Nov 21, 1997)

Here is the previous lecture

Here is the Next lecture

Back to the list of lectures

Last time, we found that there was a maximum value of m. Also, earlier, we showed that 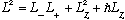, which yields

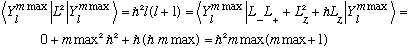
(Where I used the fact that 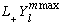=0).

Solving for m(max) gives (by inspection!) m(max)=l.

(It's a quadratic equation, but the other root is negative, which doesn't make sense for the maximum.)

Similarly, since we know 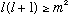, there must also be some minimum m(min), presumably negative, where 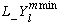=0.

This time you use in a completely analogous way to get

The solutions to this little quadratic equation are m(min)=1+l, or -l.

Clearly m(min)=l +1 > l = m(max) is nonsense, so we must have m(min)= -l.

(Note also: Since 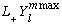=0 is a 1st order ODE (at least, after taking out the already-known phi dependence), it has only one solution, and thus there cannot be two different sets of eigenfunctions with different mmax's)

Apparently, the allowed values of m are going up in steps of 1, running from

m(min)=-l, -l+1, -l+2, ..., l-2, l-1, l = m(max)

Suppose there are a total of N steps here. Clearly, N must be an integer.

We see that -l +N = +l. So, l=N/2. Which means l must be either an integer (if N is even) or a half integer (if N is odd). These are the only possibilities, and for now we assume N is even, since 1/2 integer m's seems so bizarre.

Since there are N+1 possible different values for m, there are

2l+1 possible m values for each l.

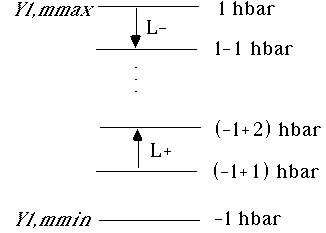
We can draw a little ladder diagram, very much like we did with the H.O. (Here, the rungs represent L_z, rather than energy, of course)

There are a total of 2l+1 "rungs" on the ladder. (count them)

We can also find the constant C required to normalize the eigenfunctions:

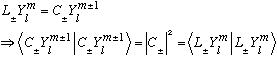
However, 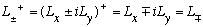. Which tells us

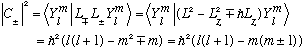
Most people choose the C's to be real and positive (but this is convention).

What have we learned?

The eigenvalues of m run from -l up to +l.

If the m's are integers, so is l .

We have found the raising and lowering operators that take us from one m state to another (even with proper normalization, using the C's.)

What about the eigenfunctions themselves? (We did the L_z part just a few pages ago (11-3) and fou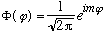nd , and .

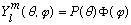But we'd still like to find the P functions. To do this requires solving a first order O.D.E.

Recall the explicit form we found at the beginning of this chapter:

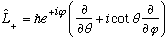
And, we know

=0=.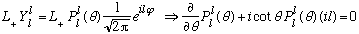

As advertised, we have a first order ODE for .

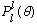I claim that works. Don't ask how I know - just check it:

.

It works! And 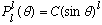is the general solution. (It's a 1st order linear ODE -> there should be only one unknown parameter, which is C).

Putting together phi and theta dependence :

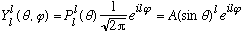
and then our lowering operators tell us that e.g.

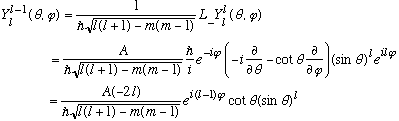
and so on.

We've got them all! (Except for the one normalization constant "A", which we need to find by direct integration. It's not super hard to normalize 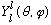. But never mind, we won't bother...)

Gas. (p 196) goes through some details to generalize this result, to give

.

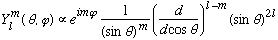(And, once we figure out A, we immediately have the normalization of all the others)

But, now that we know how to do it, it's obviously easier to go look up the darn things somewhere, rather than calculating them ourselves!

Some comments and observations:

When m=m(max)=l, <L_z> is the max possible, and

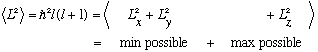
In this case,

If l is big, this function is strongly peaked at theta = pi/2, which corresponds to a particle orbiting in the x,y plane. (Max angular momentum around the z axis -> orbit is in xy plane. Makes sense! It's the correspondence principle.)

Another thing: the length of the angular momentum vector (in units of hbar) is |L| = 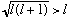. But the z projection, |L_z| is of course just m. The maximum z projection is m=l. Putting this together says:

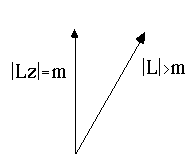
Even when the angular momentum about the z axis is maximum, it is never the case that the particle is spinning purely in the xy plane. I.e, the L vector never points "straight up". (There must always be some amount of L_x and L_z.) This is quite non-classical, where L can point any way you want.

It is consistent with our "generalized" Heisenberg uncertainty principle!

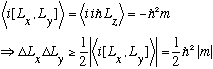
For the state we're discussing (maximum L_z, m=l), you cannot know both L_x and L_y at the same time. They obey an uncertainty relation! (It turns out that in this state of maximum L_z, you can show )

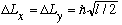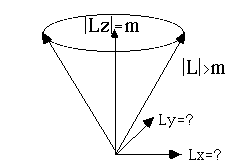
So that little picture I drew is itself not quite right, since we don't know the x or y components, the angular momentum vector must be fuzzed out in a quantum way, over some cone-like region.

Here is the Next lecture