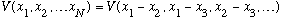Physics 3220, Fall '97. Steve Pollock.

3220 - Notes, lecture 43 (Fri, Dec 5, 1997)

Here is the previous lecture

Here is the Next lecture

Back to the list of lectures

Last time we introduced some general ideas about N-particle wavefunctions, including the form of the Hamiltonian. There is an important special case, which we will focus some attention on. It is not the only physically interesting case, but a lot of physics falls under this category. It is the case where there are no external fields, i.e. the potential is determined only by the relative positions of the particles. In this case, .

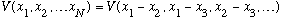For such a system, the location of the origin is quite irrelevant for the energy. Only relative positions matter.

There is an even more specialized case (it is a subcase of the above), where the potential breaks up into a sum of independent 2 particle terms, i.e.

.

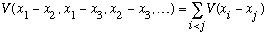Notice that in this case, which we call "2 body forces", the potential depends on the separation between each and every pair. The pairs are all treated completely symmetrically (no one is special) and there are no 3 body forces, i.e. the potential between particles 1 and 2 is completely independent of where any other particles are. This is true of many fundamental forces of nature (like gravity, or electricity), and so this special case is still extremely useful and quite general.

I claim that if there are no external fields (either of the special cases considered above) then the total momentum of the system is conserved.

Let me first show you how the classical proof of this statement goes. Then, (following the philosophy of our example in Ch. 10 where we used rotational symmetry to prove conservation of angular momentum) we will use translational symmetry to prove the statement in quantum mechanics.

Classical proof:

Define total momentum in the obvious way:

.

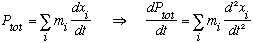Newton's second law says

.

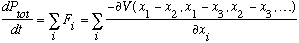We make some abbreviations:

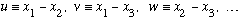
What we need for the right side of the Newton's law equation is a bunch of

partials of V with respect to x's. Changing variables to u, v, w, etc. we get:

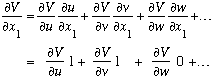
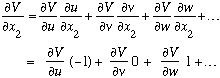
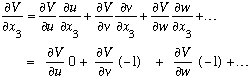
If you add these all up, you see that every term which appears in one expression always appears in another one with the negative sign, they cancel term by term and the total sum is zero! (Convince yourself that this continues to be true no matter what n is...)

We just showed ,

momentum is conserved!

Quantum proof:

(We use symmetry, similar to how we showed cons. of angular momentum.)

Observe that if there are no external forces, H is invariant under translations. That is, (for any translation of all x's)

.

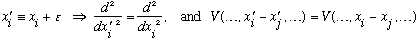Which means

.

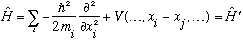Write down Schrodinger's equations in the x and x' frames

But I just showed H=H'. The energy is invariant, but the wave function is not. Let's Taylor expand u_E:

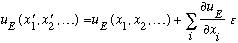
Plug this into the second equation we had just written above:

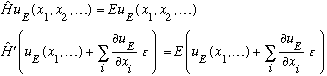
Now subtract the top from the bottom, using H=H', leaving

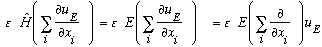
The epsilons cancel, and if we define total momentum in the most obvious way possible, namely

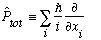
then what we just showed above is

Since this is true for any function u_E (which form a complete set), then it must be true that

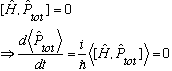
which is the quantum mechanical version of conservation of total momentum. It arises clearly and directly from invariance under translation.

This is just another example of Noether's theorem:

invariance (or symmetry) leads to a conservation law.

Next we will consider the simplest N particle system, namely N=2.

Suppose first there was no potential at all, so we are simply describing 2 non-interacting free particles. We can write down the Hamiltonian:

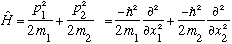
and, as usual, we are trying to solve Schrodinger's Eq, which here looks like

This is a PDE in two variables, x1 and x2. Next, we will try first separating variables (to solve it directly), and also changing variables (to center of mass, and relative) to solve it an alternative way.